Modeling of Nonlinear Interference
Analogue-digital simulation
An analogue-digital simulation engine has been developed, combining the circuit simulator LinzFrame with the commercial tool MATLAB. LinzFrame is a modular simulator with emphasis on analogue and radio frequency (RF) circuits and numerical techniques such as envelope methods. The digital baseband signal is processed entirely by MATLAB, employing its full functionality of its toolboxes. Applications include non-linear distortion analysis, digital pre-distortion techniques, coexistence analysis of mobile communication standards etc.
Accepted: SCEE 2020, the 13th International Conference on Scientific Computing in Electrical Engineering, February 16 - 20, 2020 - Eindhoven, the Netherlands
- Analogue-Digital Simulation of Radio Frequency and Baseband Circuitry via Simulator Coupling (extendend Abstract)
- Analogue-Digital Simulation of Radio Frequency and Baseband Circuitry via Simulator Coupling (Poster)
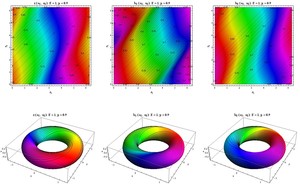
Nonlinear Scattering Operator
S-parameters defined by ratios of complex numbers describe the input-output relationships for linear devices. The assumption that the input-output relationship can be modeled by some nonlinear operator in time domain leads to the definition of a nonlinear scattering operator for nonlinear devices. This operator extends the notion of nonlinear large-signal scattering S-parameters which are defined in frequency domain in a finite dimensional setting. This nonlinear scattering operator can exists globally and X parameters are obtained as linearization of this operator as is demonstrated by the nonlinear Schrödinger equation with nonlinear Kerr point interaction.
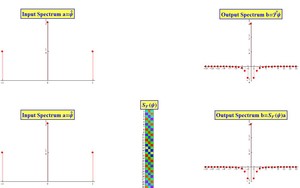
Multitone signals
Multitone signals are used as stimulus signals in nonlinear measurements of
microwave devices and circuits. Based on these measurements, models such as the third order
intercept point, X-parameters, or, in recent time, Nonlinear Scattering Operators (NSO),
are derived, the latter having their origin in quantum physics.
This document analyses the constraints on the stimulus signal for applying NSO techniques.
Specifically, the signal waveforms are limited to relative power levels, avoiding possible singularities
of the gain function in NSO calculation, implying that the scattering function does exist.
 Technische Universität Wien
Technische Universität Wien